View more examples »To complete the square when a is greater than 1 or less than 1 but not equal to 0, factor out the value of a from all other terms For example, find the solution by completing the square for 2 x 2 − 12 x 7 = 0 a ≠ 1, a = 2 so divide through by 2 2 2 x 2 − 12 2 x 7 2 = 0 2 which gives us x 2 − 6 x 7 2Algebra Calculator is a calculator that gives stepbystep help on algebra problems See More Examples »
Mcq In Math
Expand x + 2 y + 4 z whole square
Expand x + 2 y + 4 z whole square-The intersection of the two spheres is a circle perpendicular to the x axis, at a position given by x above Substituting this into the equation of the first sphere gives y 2 z 2 = 4 d 2 r 12 (d 2 r 22 r 12) 2 / 4 d 2 You might recognise this is the equation of a circle with radius h where4 Binomial Expansions 41 Pascal's riTangle The expansion of (ax)2 is (ax)2 = a2 2axx2 Hence, (ax)3 = (ax)(ax)2 = (ax)(a2 2axx2) = a3 (12)a 2x(21)ax x 3= a3 3a2x3ax2 x urther,F (ax)4 = (ax)(ax)4 = (ax)(a3 3a2x3ax2 x3) = a4 (13)a3x(33)a2x2 (31)ax3 x4 = a4 4a3x6a2x2 4ax3 x4 In general we see that the coe cients of (a x)n



Simplifying Square Root Expressions Video Khan Academy
Jan , 17Explanation Expansion of (a b)n gives us (n 1) terms which are given by binomial expansion xnCra(n−r)br, where r ranges from n to 0 Note that powers of a and b add up to n and in the given problem this n = 5 In (x − 3y)5, we need coefficient of x3y2, we have 3rd power of x and as such r = 5 −3 = 2 and as such the desiredExercise 3 Expand the following expression, writing your answer in its simplest form Be careful of notation and do not use spaces in your answer ( x ) 2 = x2 xMathematics Menu The following are algebraix expansion formulae of selected polynomials Square of summation (x y) 2 = x 2 2xy y 2 Square of difference (x y) 2 = x 2 2xy y 2 Difference of squares
= ( Σ (xμ)²Integrate 1/(cos(x)2) from 0 to 2pi;May 29, 18Transcript Example Expand (4a – 2b – 3c)2 (4a – 2b – 3c)2 = (4a (–2b) (–3c)) 2 Using (x y z)2 = x2 y2 z2 2xy 2yz 2zx Putting x = 4a
59 = 625 Given, ab bc ca = 59 a 2 b 2 c 2 118 = 625 a 2 b 2 c 2 118 – 118 = 625 – 118 subtracting 118 from both the sides Therefore, a 2 b 2 c 2 = 507 Thus, the formula of square of a trinomial will help us to expand 7th Grade Math Problems 8th Grade Math PracticeStatistics Alternate variance formulas Sal explains a different variance formula and why it works!Expand Using the Binomial Theorem (XY)^4 Use the binomial expansion theorem to find each term The binomial theorem states Expand the summation Simplify the exponents for each term of the expansion Simplify each term Tap for more steps Multiply by Anything raised to is


Square Root Of 2 Wikipedia


Solution Find The Term Involving X 2 In The Expansion Of X 3 K X 10
Free Complete the Square calculator complete the square for quadratic functions stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie PolicyX3=5 1/3 1/4 y=x^21 Disclaimer This calculator is not perfect Please use at your own risk, and please alert us if something isn't working Thank youStudents trying to do this expansion in their heads tend to mess up the powers But this isn't the time to worry about that square on the xI need to start my answer by plugging the terms and power into the TheoremThe first term in the binomial is x 2, the second term in 3, and the power n is 6, so, counting from 0 to 6, the Binomial Theorem gives me



Example 21 Factorize 4x2 Y2 Z2 4xy 2yz 4xz Examples


Rectangles Geom Raster Ggplot2
A 2 b 2 c 2 2 ×Factorization of x 3 y 3 It can be seen in most book that x 3 y 3 can be factorized by dividing the expression by (x y) After division we get a quotient of (x 2 xy y 2) with no remainder Therefore However, this method involves knowing the factor (x y) beforehand (and the understanding of Factor Theorem)The Derivative Calculator will show you a graphical version of your input while you type Make sure that it shows exactly what you want Use parentheses, if necessary, e g a/ (bc)


Ambiguous Pemdas



Expand Using Identities 3x 5y 2
Steps for Solving Linear Equation y = 3x 4 y = 3 x 4 Swap sides so that all variable terms are on the left hand side Swap sides so that all variable terms are on the left hand side 3x4=y 3 x 4 = y Subtract 4 from both sides Subtract 4 from both sidesPutting it all together, the steps are as follows ( x3 ) ( x4) = ( xxx ) ( xxxx) = xxxxxxx = x7 Then the simplified form of (x3) (x4) is x7 Note that x7 also equals x(34) This demonstrates the first basic exponent rule Whenever you multiply two terms withExpand (x 2y z) 2 Solution (x 2y z) 2 is in the form of (a b c) 2 Comparing (a b c) 2 and (x 2y z) 2, we get a = x b = 2y c = z Write the formula / expansion for (a b c) 2 (a b c) 2 = a 2 b 2 c 2 2ab 2bc 2ac Substitute x for a, 2y for b and z for c (x 2y z) 2



Numbers In Python Real Python



The Factors Of X 2 4y 2 4y 4x Y 2x 8 Are A X 2y 4 X 2y 2 B X Y 2 X 4y 4 C Youtube
1 Identify the terms, their coefficients for each of the following expressions In the expression 5xyz 2 – 3zy, the terms are 5xyz 2 and –3zy coefficient of xyz 2 in the term 5xyz 2 is 5 coefficient of zy in the term –3zy is –3 In the expression 1 x x 2, the terms are 1, x and x 2Expand following, using suitable identities (x 2y 4z) 2 Advertisement Remove all ads Solution Show Solution It is known that, (x y z) 2 = x 2 y 2 z 2 2xy 2yz 2zx (x 2y 4z) 2 = x 2 (2y) 2 (4z) 2 2(x)(2y) 2(2y)(4z) 2(4z)(x) = x 2 4y 2 16z 2Click here 👆 to get an answer to your question ️ Expand (x2y3z)²
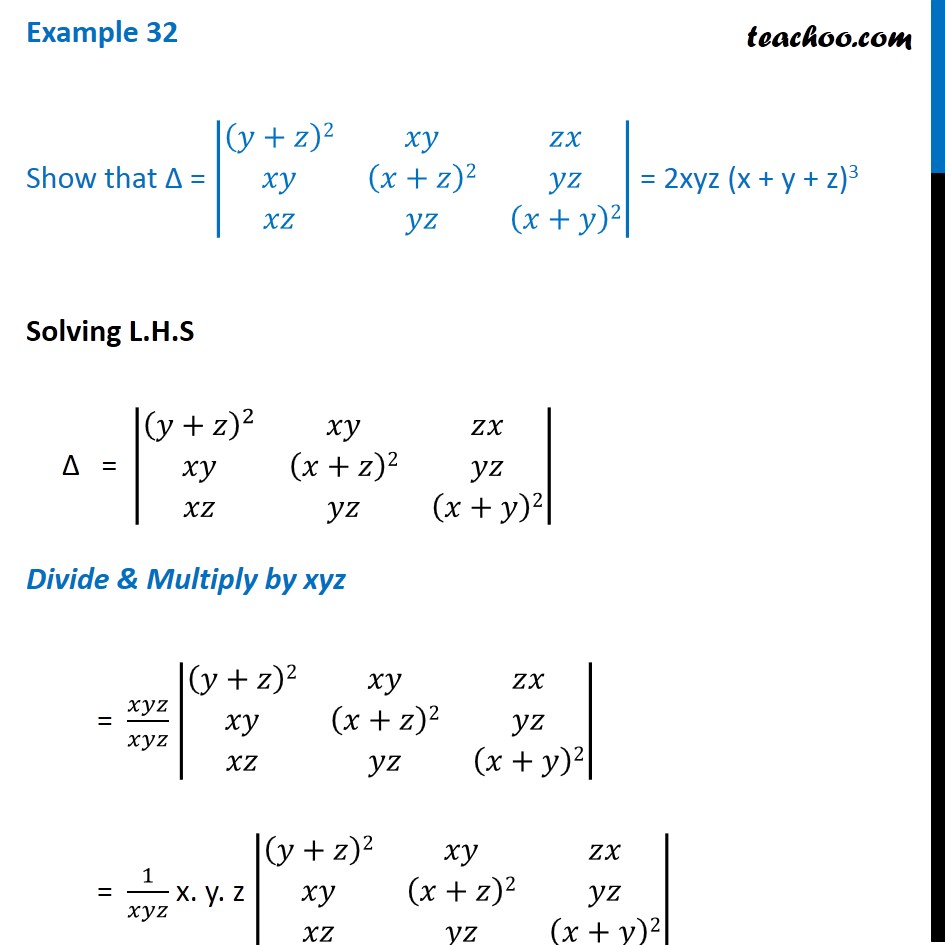


Example 32 Show That Determinant 2xyz X Y Z 3 Class 12


Three Dimensional Space Wikipedia
I√2/2, where the pluses and minuses can be taken in any order They are the four points at the intersections of the diagonal lines y = x and y = x with the unit circle We'll see them later as square roots ofThe calculator will find the binomial expansion of the given expression, with steps shown Show Instructions In general, you can skip the multiplication sign, so 5 x is equivalent to 5 ⋅ x In general, you can skip parentheses, but be very careful e^3x is e 3 x, and e^ (3x) is e 3 x Also, be careful when you write fractions 1/x^2 ln (xFor a population, the variance is calculated as σ²



Expand Using The Identity A 2x 3y Z 2 Brainly In
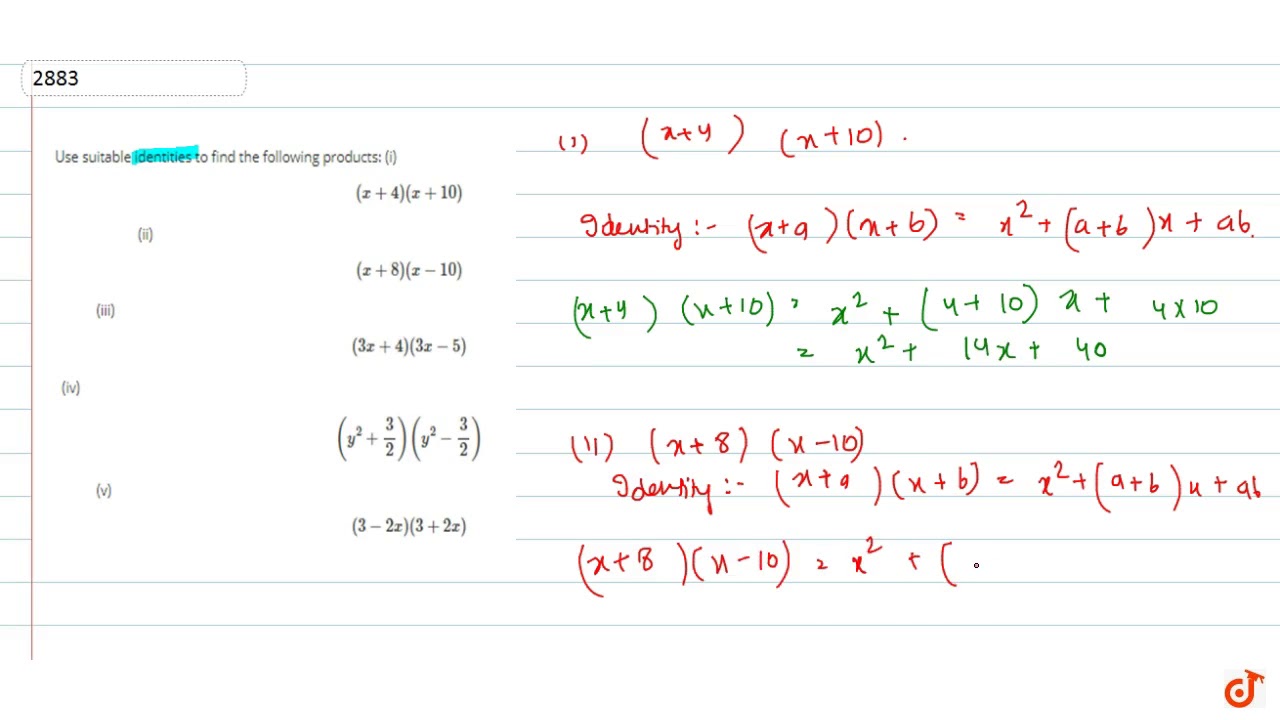


Use Suitable Identities To Find The Following Products I X 4 X 10 Ii X 8 X 10 Ii Youtube
Isaac Newton wrote a generalized form of the Binomial Theorem However, for quite some time Pascal's Triangle had been well known as a way to expand binomials (Ironically enough, Pascal of the 17th century was not the first person to know aboutAnd now solve the difference of two squares with a = 36 and b = 4y 2 Solution Factor the equation (rearranged) 36 − 4 y 2 using the identity a 2 − b 2 = ( a b) ( a − b) First factor out the GCF 4 ( 9 − y 2) Both terms are perfect squares so from a 2 b 2 we can find a and bTo simplify your expression using the Simplify Calculator, type in your expression like 2(5x4)3x The simplify calculator will then show you the steps to help you learn how to simplify your algebraic expression on your own Typing Exponents Type ^ for exponents like x^2 for x squared



Simplifying Square Root Expressions Video Khan Academy
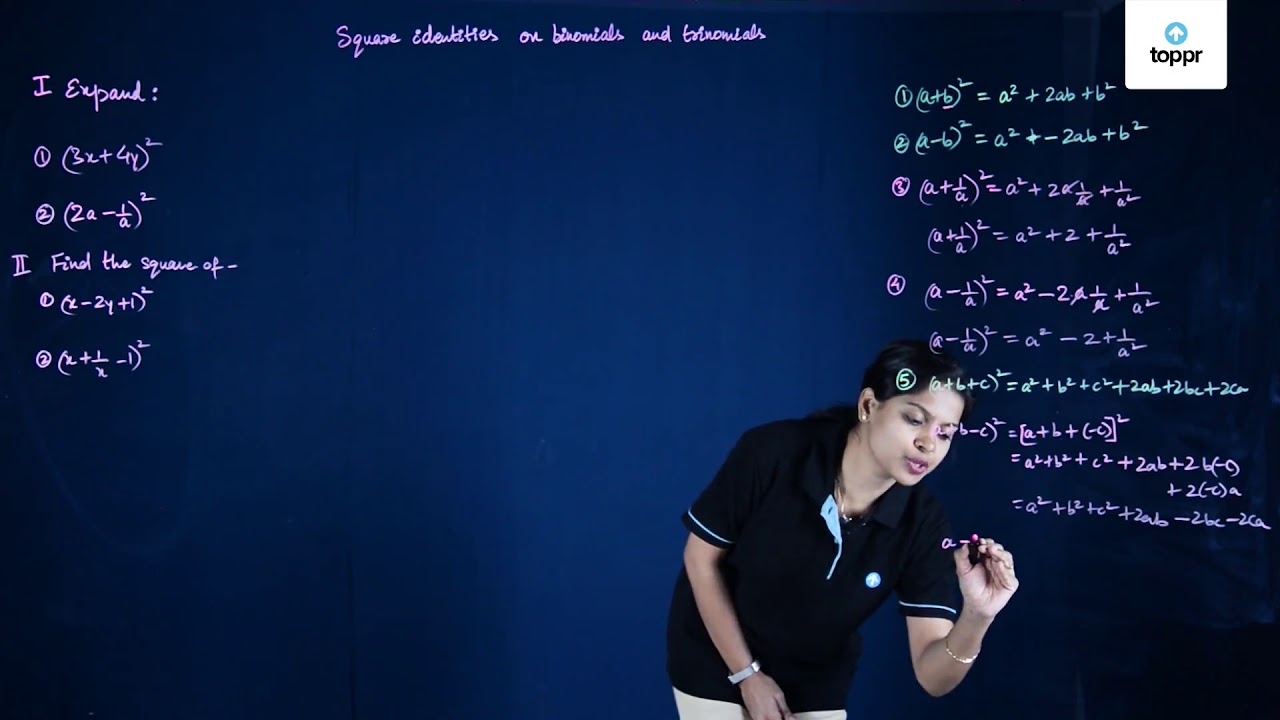


Standard Identities Of Binomials And Trinomials Equations Examples
= ( (Σ x²) / N ) μ²The procedure to use the binomial expansion calculator is as follows Step 1 Enter a binomial term and the power value in the respective input field Step 2 Now click the button "Expand" to get the expansion Step 3 Finally, the binomial expansion will be displayed in the new windowMar 16, 17Ex 25, 4 Expand each of the following, using suitable identities (x 2y 4z)2 (x 2y 4z)2 Using (a b c)2 = a2 b2 c2 2ab 2bc 2ac Where a = x , b = 2y, c = 4z = x2 (2y)2 (4z)2 2(x) (2y) 2(2y) (4z) 2 (4z) (x) = x2 4y2 16z2 4xy 16yz 8xz Ex 25, 4 Expand each of the following, using suitable identities (ii) (2x y z)2 (2x y z)2 = (2x ( y) z)2 Using (a b c)2 = a2 b2 c2 2ab 2bc 2ac Where a = 2x , b = y, c = z = (2x)2 (y)2 (z)2 2



The Binomial Theorem Fractional Powers Expanding 1 2x 1 3 Youtube


If Z Is A Complex Number Then Is Z 2 Equal To Mod Z 2 Quora
Proving the Expansion of a plus b Whole Square Geometrically In this section, we are going to see, how to prove the expansion of (a b) 2 geometrically We can prove the the expansion of (a b) 2 using the area of a square as shown below Solved Problems Problem 1 Expand (x y) 2Access instant learning tools Get immediate feedback and guidance with stepbystep solutions and Wolfram Problem Generator LearnJan 24, 18You can't, really While it looks like you can just cancel out the squares with the square root, this only works if you have one square within the radical Since we're adding two together, it's slightly different There's no way to factor x^2y^2 to get a perfect square The only real way to simplify this expression is by using math that's at a higher than algebra level, so for all
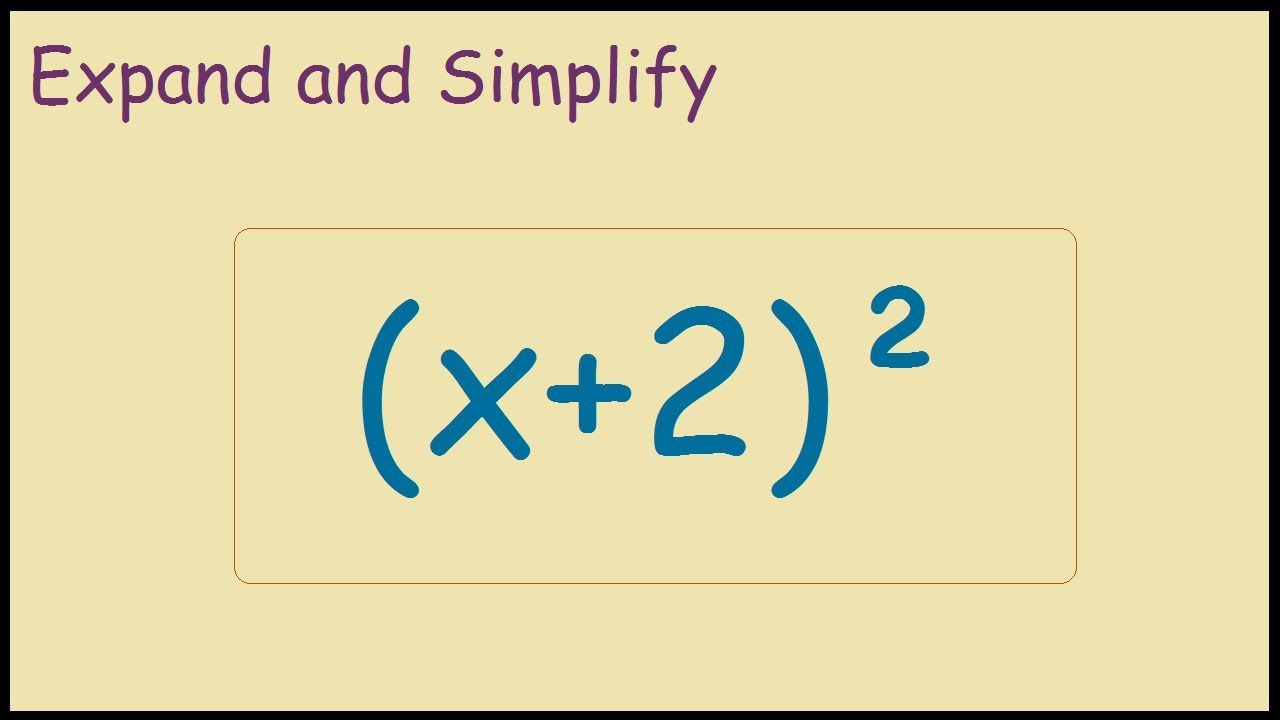


X 2 2 Expand And Simplify Using Foil Method Youtube



Expand X By 2 Y 1 By 4 Whole Square Brainly In
It is 1/4*base2*(vertical height)2 in units of length to the fourth power If the lengths of the sides are X, Y and Z, then the area squared is (X Y Z)*( X Y Z)*(X Y Z)*(X Y Z)/16Enter the function you want to differentiate into the Derivative Calculator Skip the f (x) = part!Expand (x 2 3) 6;


Covid Economics Centre For Economic Policy Research



The Term Independent Of X In Expansion Of X 1 X 2 3 X 1
In algebra, a quadratic equation (from the Latin quadratus for square) is any equation that can be rearranged in standard form as = where x represents an unknown, and a, b, and c represent known numbers, where a ≠ 0If a = 0, then the equation is linear, not quadratic, as there is no term The numbers a, b, and c are the coefficients of the equation and may be distinguished by callingExpand each of the following using suitable identities X 2 Y 4 Z whole square Get the answers you need, now!Factorization goes the other way suppose we have an expression that is the difference of two squares, like x²25 or 49x²y², then we can factor is using the roots of those squares For example, x²25 can be factored as (x5)(x5) This is an extremely useful method that is used throughout math



Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9



Variance Wikipedia
) / N Another equivalent formula is σ²Expand (xy)^4 Use the Binomial Theorem Simplify each term Tap for more steps Rewrite using the commutative property of multiplication Multiply by Apply the product rule to Rewrite using the commutative property of multiplication Raise to the power of Multiply byIn algebra, a nested radical is a radical expression (one containing a square root sign, cube root sign, etc) that contains (nests) another radical expression Examples include 5 − 2 5 , {\displaystyle {\sqrt {52 {\sqrt {5}}\ }},} which arises in discussing the



Scalable And Isotropic Expansion Of Tissues With Simply Tunable Expansion Ratio Park 19 Advanced Science Wiley Online Library



Expand And Simplify Binomial Squares 2x 3y 2 Youtube
The hyperbolic functions represent an expansion of trigonometry beyond the circular functionsBoth types depend on an argument, either circular angle or hyperbolic angle Since the area of a circular sector with radius r and angle u (in radians) is r 2 u/2, it will be equal to u when r = √ 2In the diagram, such a circle is tangent to the hyperbola xy = 1 at (1,1)Multiply as we do multiplication of two binomials and we get = a (a b) b (a b) = a 2 ab ab b 2 Add like terms and we get = a 2 2ab b 2 Rearrange the terms and we get = a 2 b 2 2ab Hence, in this way we obtain the identity ie (a b) 2 = a 2 b 2 2ab Following are few applications this identityIntegrate x^2 sin y dx dy, x=0 to 1, y=0 to pi;


A Cancer Rainbow Mouse For Visualizing The Functional Genomics Of Oncogenic Clonal Expansion Nature Communications


Fmri Data Analysis Using Spm Springerlink
A complex number z = x yi will lie on the unit circle when x 2 y 2 = 1 Some examples, besides 1, –1, i, and –1 are ±√2/2 ±Log b (x y) = y ∙ log b (x) For example log 10 (2 8) = 8∙ log 10 (2) Derivative of natural logarithm The derivative of the natural logarithm function is the reciprocal function When f (x) = ln(x) The derivative of f(x) is f ' (x) = 1 / x Integral of natural logarithm The integral of the natural logarithm function is given byFirst remove a common factor Then factor the difference of two squares e) 32 m2n − 50 n3 = 2n(16m2 − 25n2) = 2n(4m 5n) (4m − 5n) The entire figure on the left is a square on side a The square b2 has been inserted in the upper left corner, so that the shaded area is the difference of the two squares, a2 − b2


Taylor Series Wikipedia



How To Expand Using The Identity A B C 2 B2 C2 2ab 2bc 2ca Youtube
Two numbers r and s sum up to 4 exactly when the average of the two numbers is \frac{1}{2}*4 = 2 You can also see that the midpoint of r and s corresponds to the axis of symmetry of the parabola represented by the quadratic equation y=x^2BxCIf we expand (ab)(ab) we will get a²b²Polynomial Identities When we have a sum (difference) of two or three numbers to power of 2 or 3 and we need to remove the brackets we use polynomial identities (short multiplication formulas) (x y) 2 = x 2 2xy y 2 (x y) 2 = x 2 2xy y 2 Example 1 If x = 10, y = 5a



Mcq In Math
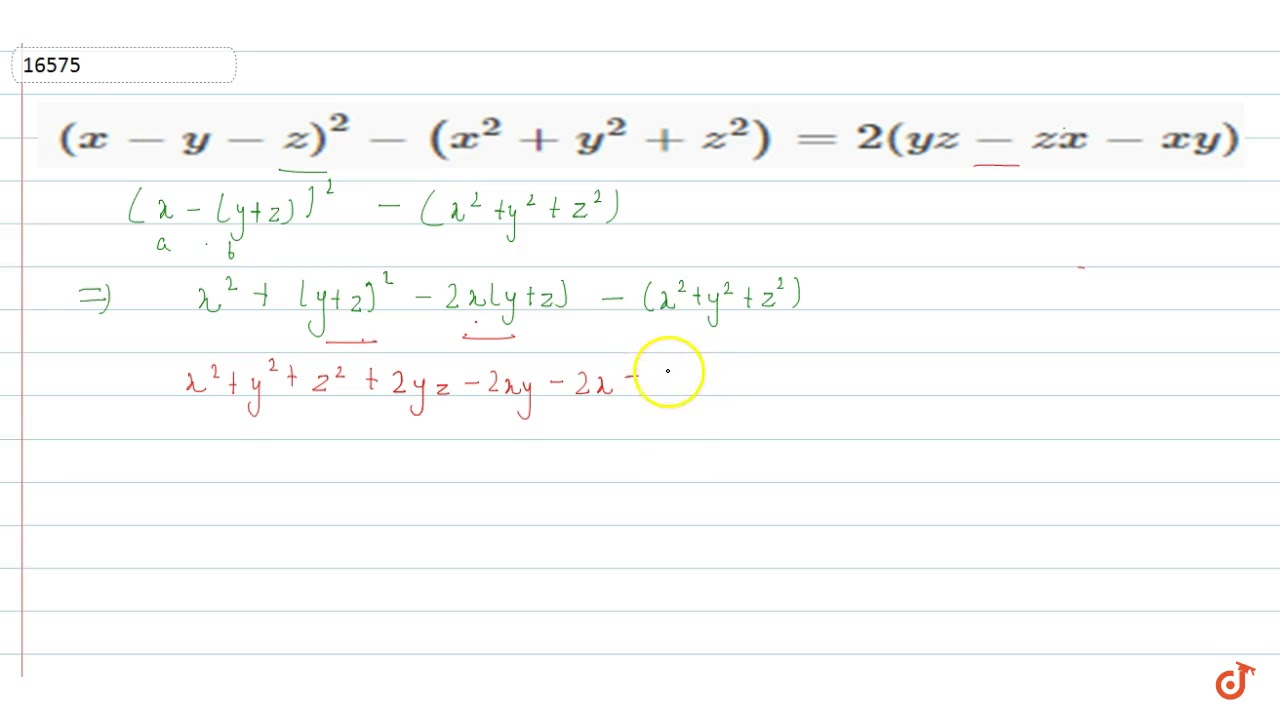


X Y Z 2 X 2 Y 2 Z 2 2 Yz Zx Xy Youtube
If we need to calculate variance bySimplify calculator Expand and simplify equations stepbystep This website uses cookies to ensure you get the best experience By using thisIntegrate x/(x1) integrate x sin(x^2) integrate x sqrt(1sqrt(x)) integrate x/(x1)^3 from 0 to infinity;
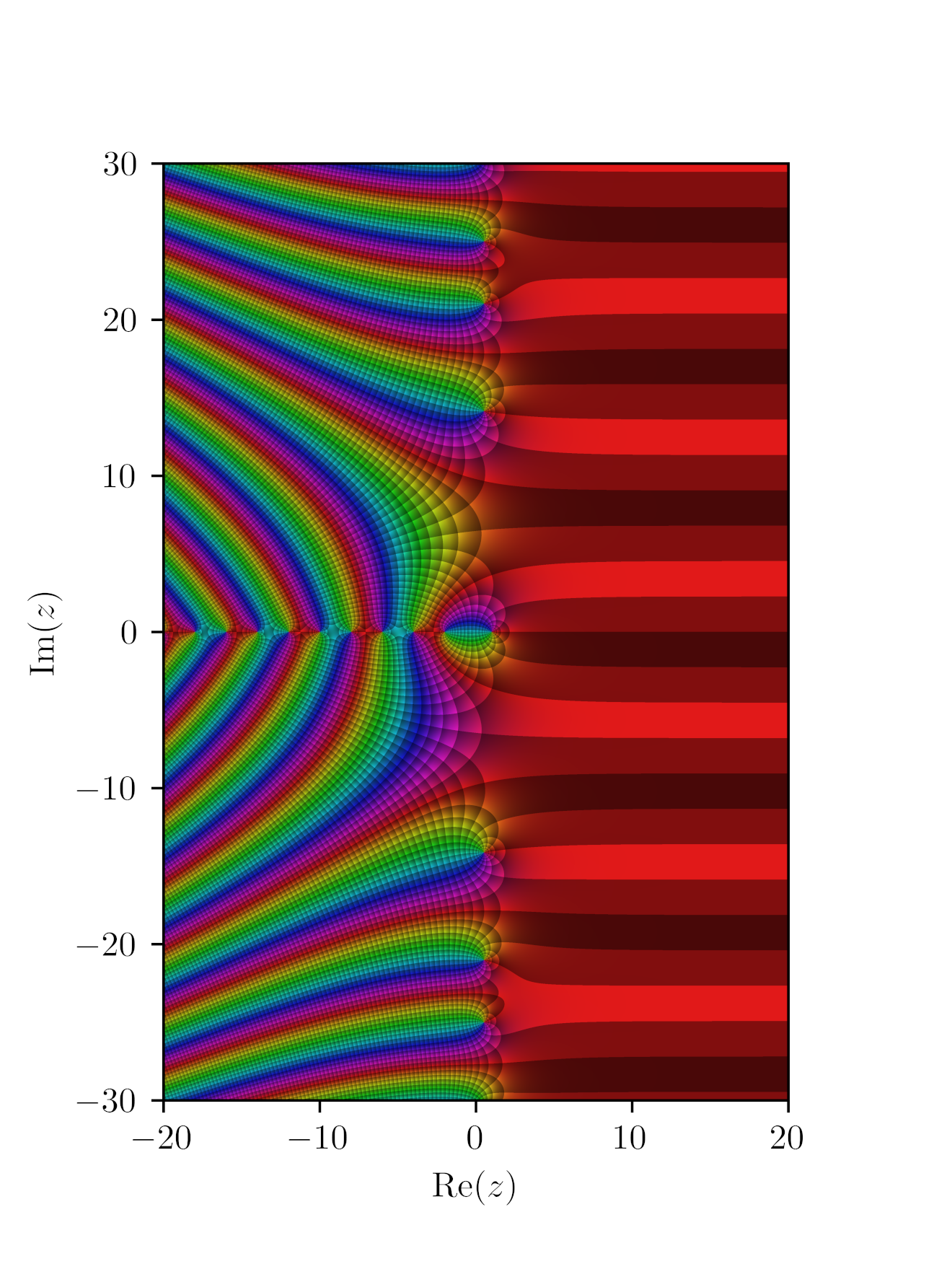


Riemann Zeta Function Wikipedia


Sketch The Interface
Enter your queries using plain English To avoid ambiguous queries, make sure to use parentheses where necessary Here are some examples illustrating how to ask about factoring factor quadratic x^27x12 expand polynomial (x3) (x^35x2) GCD of x^42x^39x^246x16 with x^48x^325x^246x16 quotient of x^38x^217x6 with x3CORRECTION Answer b is incorrect the correct answer is 4a^2 12a9Thanks Aditi JainAlgebraic Identities https//wwwyoutubecom/watch?v=HQWggFJVmKQ&list=PLExpand and simplify an expression The calculator allows you to expand and collapse an expression online , to achieve this, the calculator combines the functions collapse and expand For example it is possible to expand and reduce the expression following ( 3 x 1) ( 2 x 4), The calculator will returns the expression in two forms expanded


Space And Meaning Tobias Bernard S Gnome Blog



Find The Coefficient Of X 6y 3in The Expansion Of X 2y



The Binomial Theorem Explained With A Special Splash Of Pascal S By Brett Berry Math Hacks Medium



X Y Z Whole Square Formula A Pictures Of Hole 18



Product X 2 2y X 2 4 Xy 4y 2 Brainly In



X Square Minus Y Square Whole Cube Y Square Minus Z Square Whole Cube Z Square Minus Y Square Brainly In
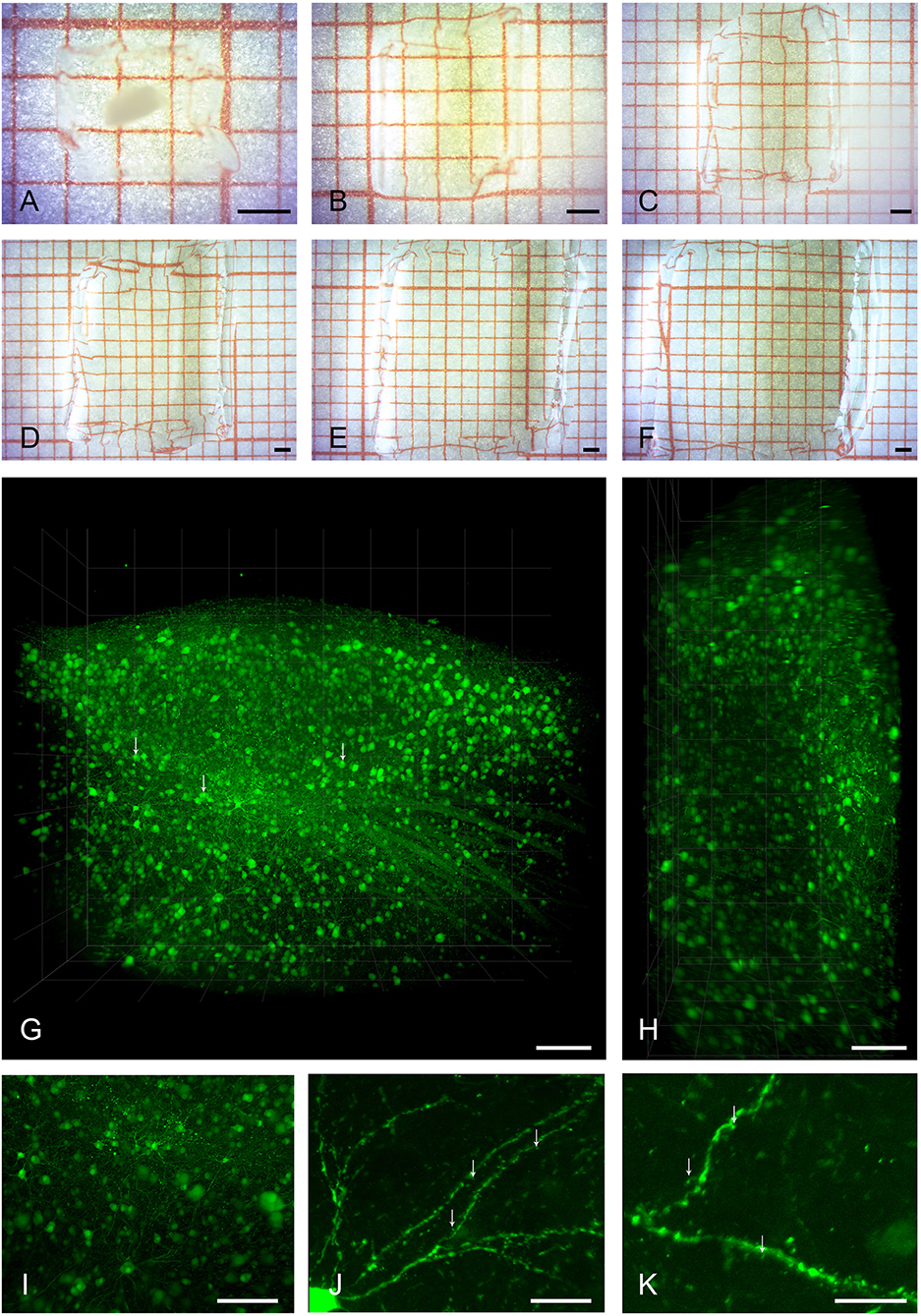


Frontiers Expansion Light Sheet Microscopy Resolves Subcellular Structures In Large Portions Of The Songbird Brain Frontiers In Neuroanatomy



Square Algebra Wikipedia



Expand X 2 Y 1 4 2 Brainly In



Rd Sharma Solutions For Class 7 Maths Chapter 7 Algebraic Expressions Download Free Pdf
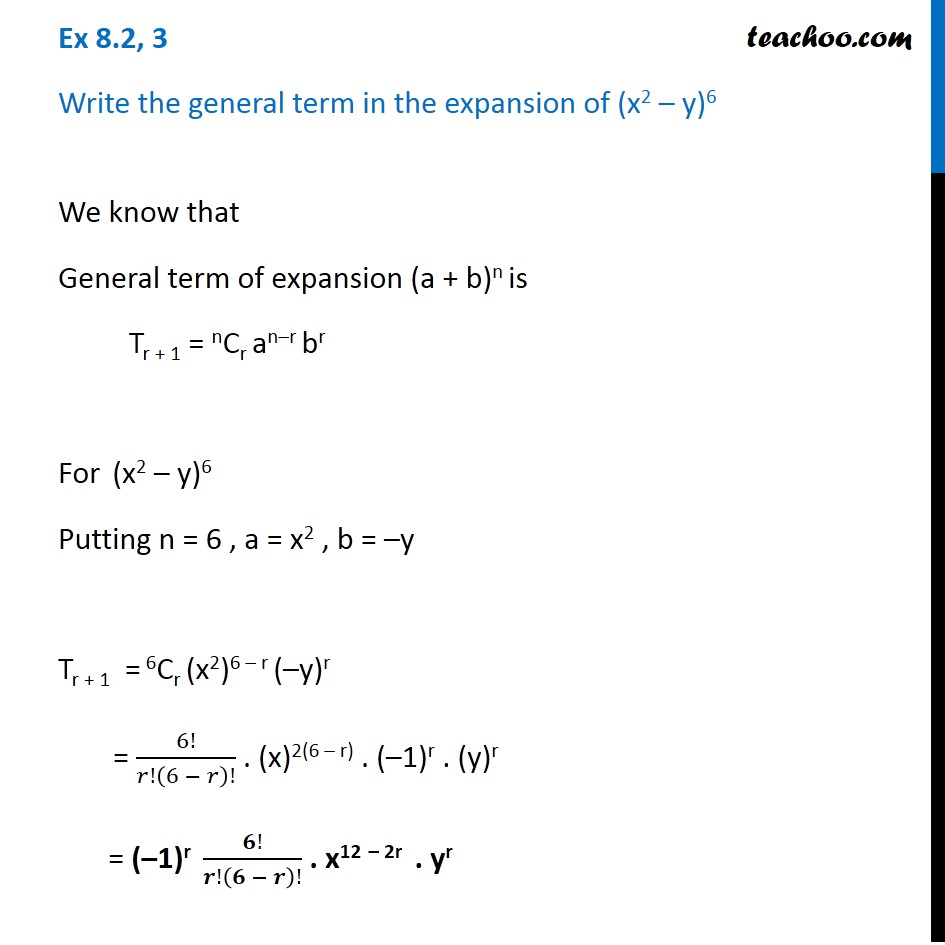


Ex 8 2 3 Write General Term In X2 Y 6 Chapter 8 Ex 8 2



Write The Coefficient Of Y In The Expansion Of 5 Y 2 Brainly In



Find A If The Coefficients Of X 2and X 3in The Expansion Of



Scalable And Isotropic Expansion Of Tissues With Simply Tunable Expansion Ratio Park 19 Advanced Science Wiley Online Library



Expand 2x 3y 2z 2 Brainly In


Ambiguous Pemdas



The Sum Of The Co Efficients Of All The Even Powers Of X In The Expansion Of 2x 2 3x 1 11 Is



2 X Square Y Square 8 Z Square 2 Root 2 X Y 4 Root 2 Y Z 8 X Z Brainly In



If Number Of Terms In The Expansion Of X 2y 3z N Are 45 Th



Factoring Using The Difference Of Squares Pattern Video Khan Academy



Find The Value Of X Cube Y Cube Z Cube Minus 3 X Y Z If X Square Y Square Z Square Is Equal To Brainly In


Expand And Simplify Binomial Squares 2x 3y 2 Youtube


Least Common Multiple Of Polynomials Video Khan Academy



Algebra Formulas For Class 10 Download Algebraic Identities For Class 10 Pdf
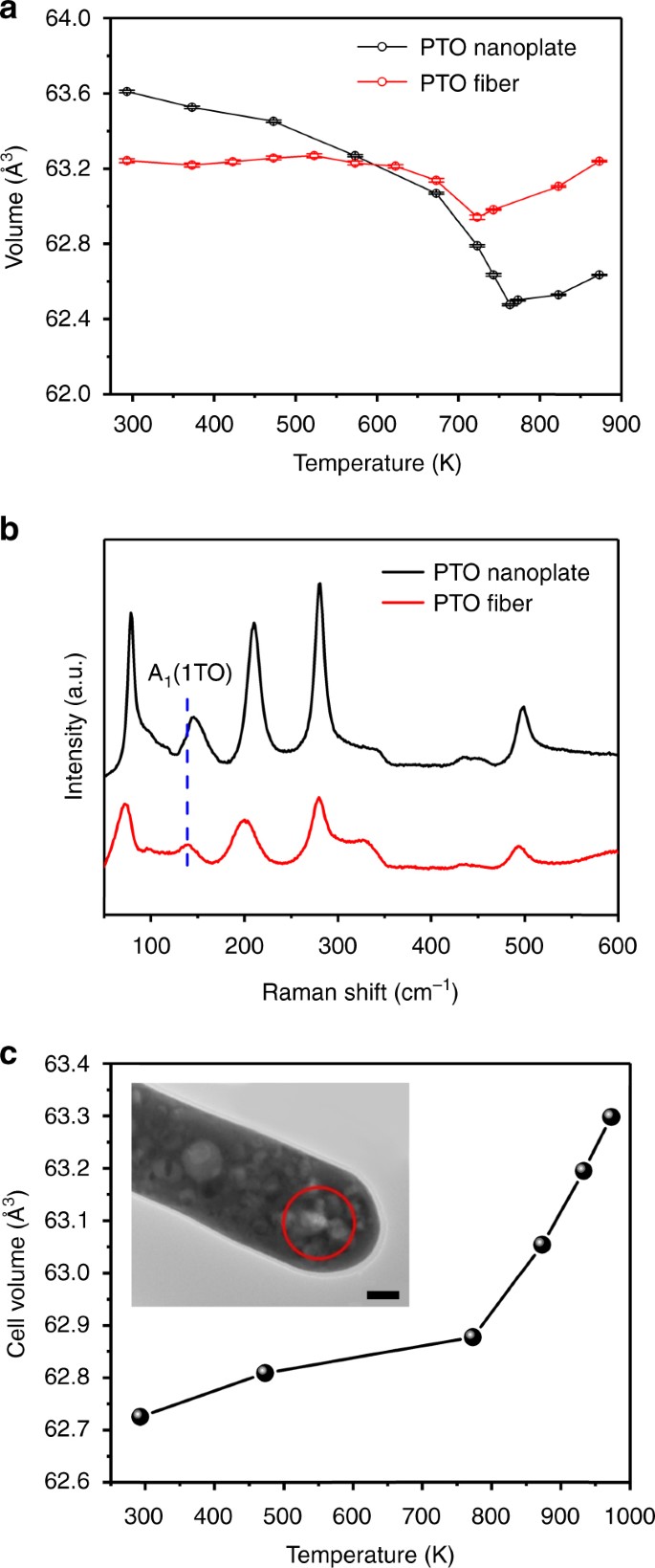


Mesopores Induced Zero Thermal Expansion In Single Crystal Ferroelectrics Nature Communications
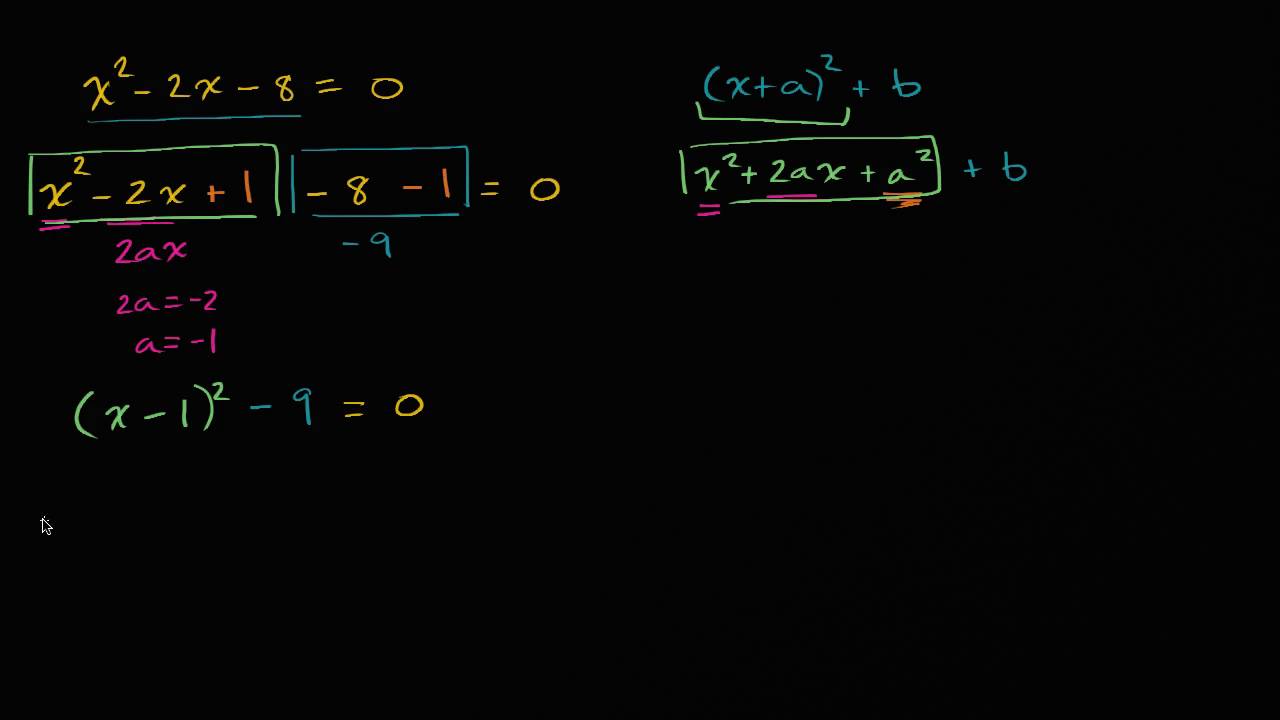


Worked Example Rewriting Solving Equations By Completing The Square Video Khan Academy



Active Noise Attenuation In Ventilation Windows The Journal Of The Acoustical Society Of America Vol 130 No 1



Example 19 Write 3a 4b 5c 2 In Expanded Form Examples


Tools Vectornator Learn



Expand 1 X X 2 3


Ambiguous Pemdas



Ex 2 5 4 Expand Each Of The Following Using Suitable



Ex 2 5 4 Expand Each Of The Following Using Suitable



Using Binomial Theorem Write Down The Expansion Of 2x 3y 5



X Y Whole Square X Y Whole Square Brainly In



Multipole And Multimode Engineering In Mie Resonance Based Metastructures


Rectangles Geom Raster Ggplot2



Partial Fraction Expansion Repeated Factors Video Khan Academy
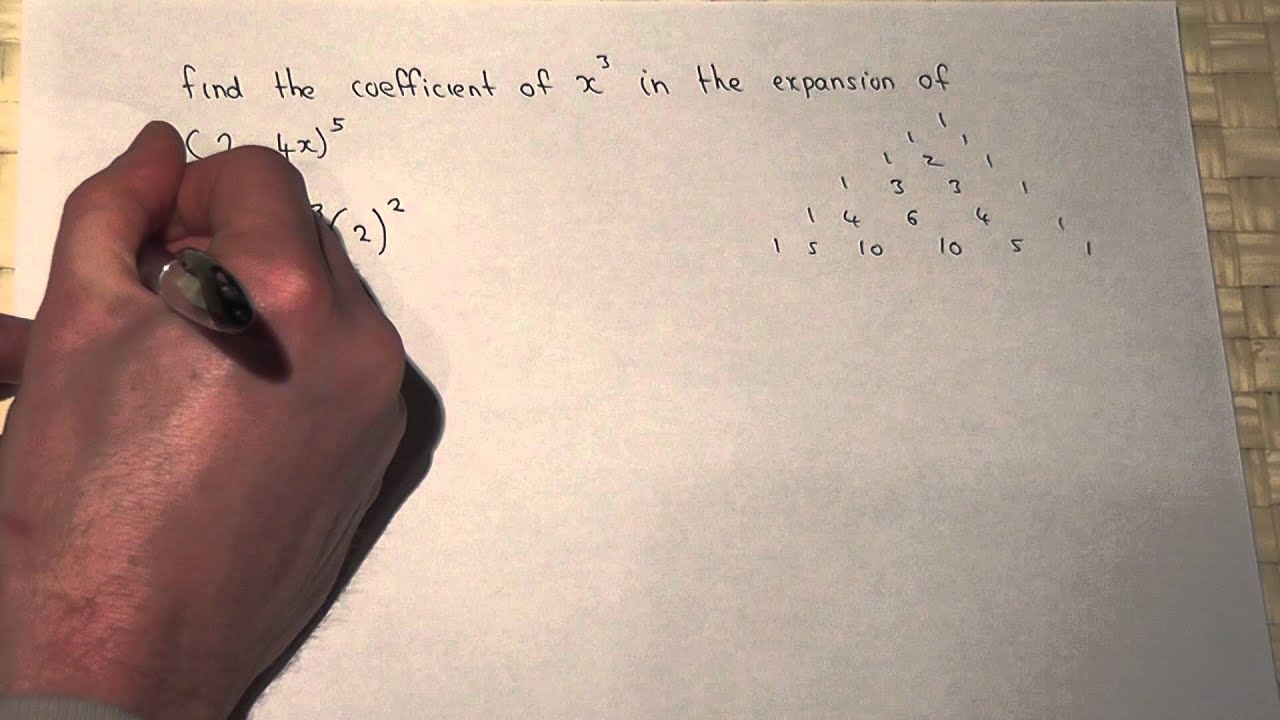


Binomial Theorem Finding The Coefficient Of X 3 In 2 4x 5 Youtube



Ex 2 5 4 Expand Each Of The Following Using Suitable


How Did Urban Land Expand In China Between 1992 And 15 A Multi Scale Landscape Analysis



Ex 2 5 4 Expand Each Of The Following Using Suitable



Perfect Square Factorization Intro Video Khan Academy
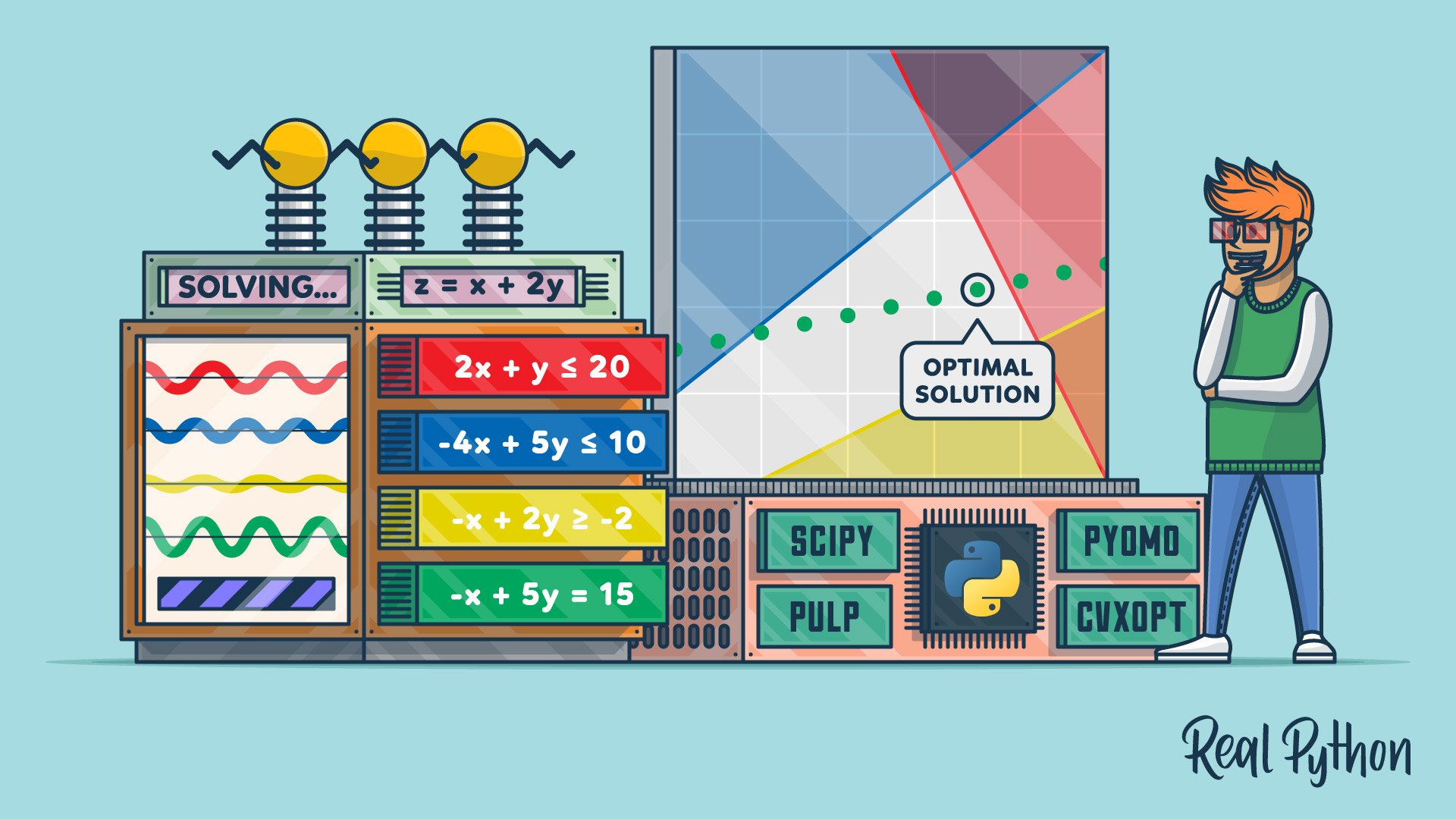


Hands On Linear Programming Optimization With Python Real Python
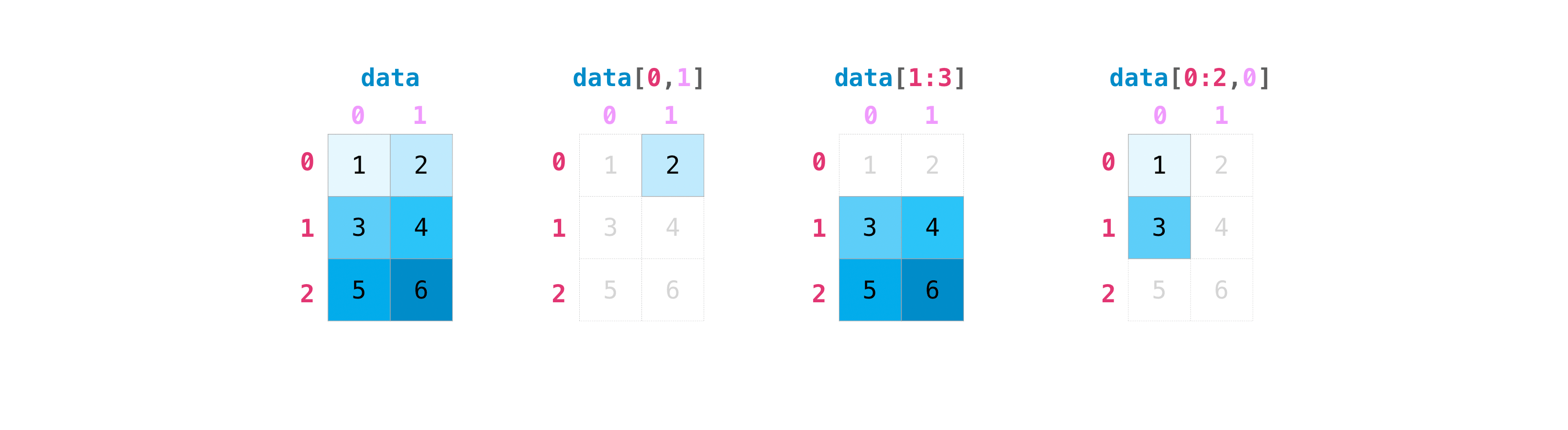


Numpy The Absolute Basics For Beginners Numpy V1 21 Dev0 Manual
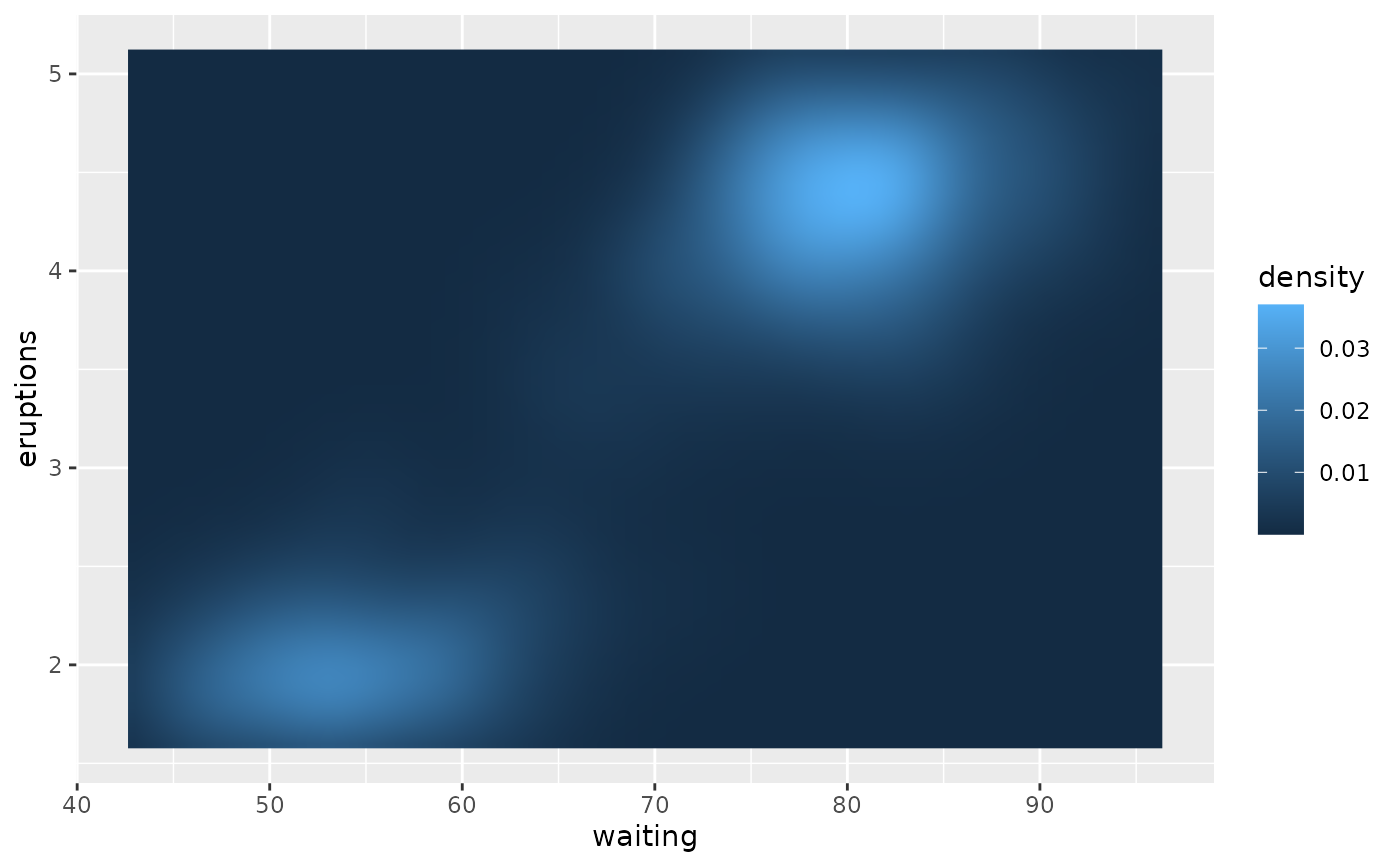


Rectangles Geom Raster Ggplot2
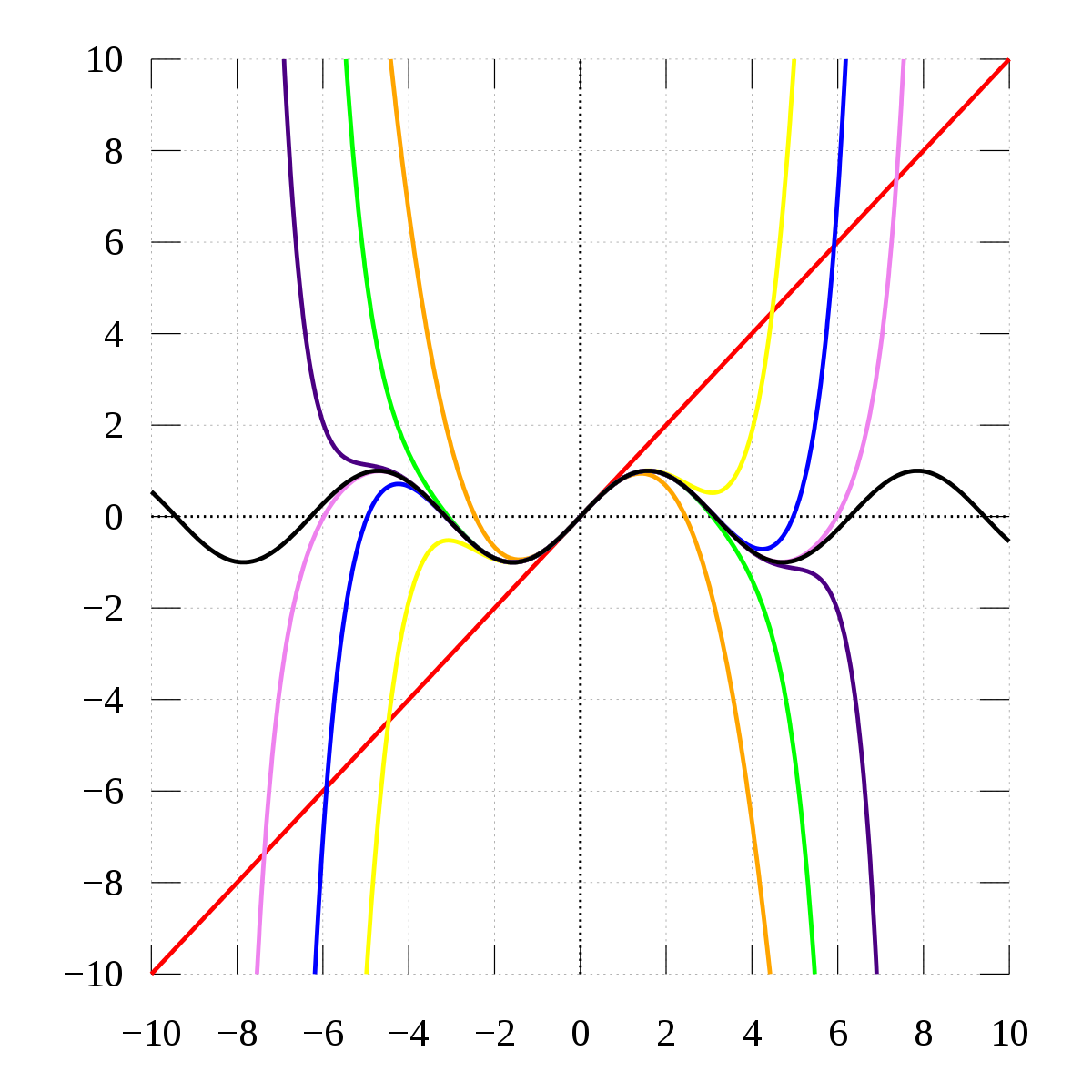


Taylor Series Wikipedia



Factorise X Square 2xy Y Square Z Square Brainly In



Expand Form Of X Y Z Whole Square Brainly In



Example 7 Find Coefficient Of X6y3 In Expansion X 2y 9


Rectangles Geom Raster Ggplot2



A Guide To Elegant Tiled Heatmaps In R 19 Rmf
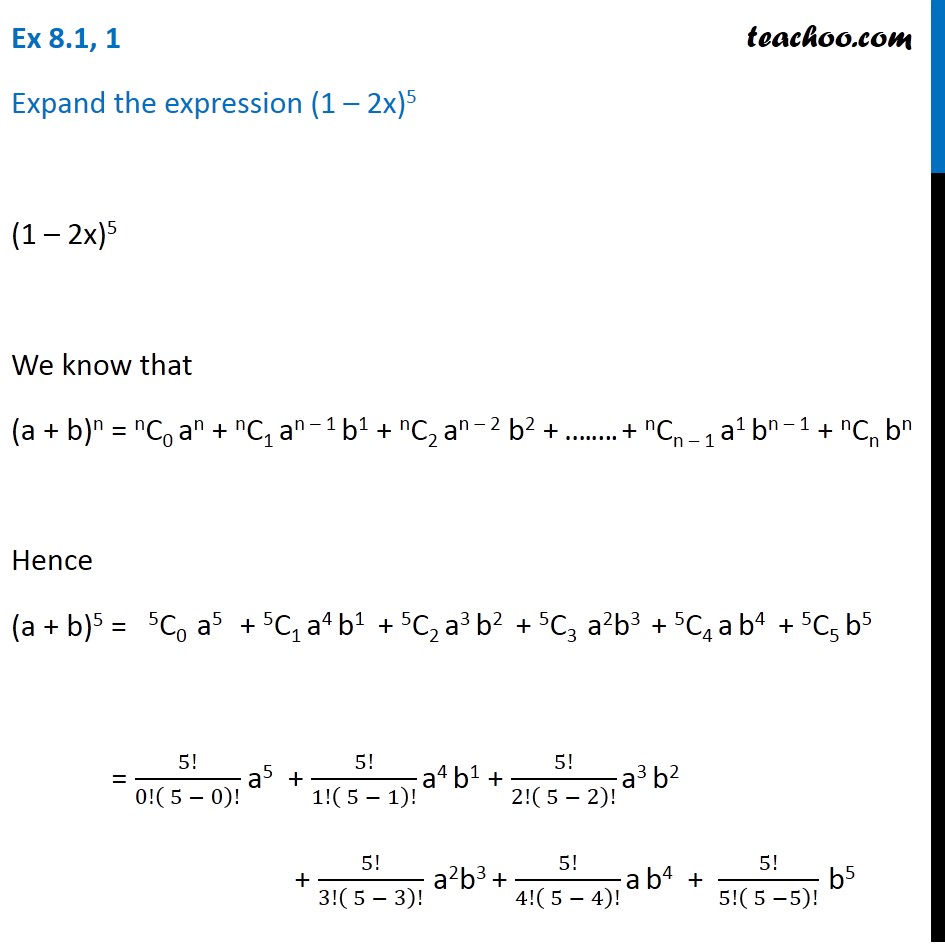


Ex 8 1 1 Expand 1 2x 5 Chapter 8 Class 11 Binomial



0 件のコメント:
コメントを投稿